FP 之 Point-free | 點燈坊

Curry Function 最主要的目的在於 Function Composition，所以儘管是 多 個 Argument，最後也可變成多個 單一 Argument 的 Function 方便組合；而 Point-free 正是最適合 Function Composition 寫法。

Version

macOS Mojave 10.15.2
VS Code 1.41.1
Quokka 1.0.276
F# 4.7
ECMAScript 2015
Ramda 0.26.1

FSharp

由於 F# 是純 FP 語言，function 可自動成為 curry function，常發現 F# 的 function 會如此設計。

List.map()
mapping : ('T -> 'U) -> list : 'T list -> 'U list

List.filter()
predicate : ('T -> bool) -> list : 'T list -> 'T list

List.reduce()
reduction : ('T -> 'T -> 'T) -> list : 'T list -> 'T

僅管 List.map()、 List.filter() 與 List.reduce() 三個 function 功能都不同，input 與 return 值也不同，但最後一個 argument 一定都是 list : 'T list。

Pipeline

let mapSquare = List.map (fun x -> x * x)
let filterOdd = List.filter (fun x -> x % 2 = 1)
let sum = List.reduce (fun a x -> a + x)

let calculate arr = 
  arr
  |> mapSquare
  |> filterOdd
  |> sum
    
calculate [1 .. 3] |> printf "%A"

第 1 行

let mapSquare = List.map (fun x -> x * x)
let filterOdd = List.filter (fun x -> x % 2 = 1)
let sum = List.reduce (fun a x -> a + x)

List.map()、List.filter() 與 List.reduce() 都僅提供 1 個 argument，所以 mapSquare()、filterOdd() 與 sum() 都是 function。

第 5 行

let calculate arr = 
  arr
  |> mapSquare
  |> filterOdd
  |> sum

將 mapSquare()、filterOdd() 與 sum() 透過 pipeline 處理 arr。

因為 mapSquare()、filterOdd() 與 sum() 的最後一個 argument 都是 list : 'T list，在 pipeline 時，F# 允許省略之。

|> 為 F# 的 pipeline，由左至右

Function Composition

let mapSquare = List.map (fun x -> x * x)
let filterOdd = List.filter (fun x -> x % 2 = 1)
let sum = List.reduce (fun a x -> a + x)

let calculate = mapSquare >> filterOdd >> sum
    
calculate [1 .. 3] |> printf "%A"

第 1 行

let mapSquare = List.map (fun x -> x * x)
let filterOdd = List.filter (fun x -> x % 2 = 1)
let sum = List.reduce (fun a x -> a + x)

List.map()、List.filter() 與 List.reduce() 都僅提供 1 個 argument，所以 mapSquare()、filterOdd() 與 sum() 都是 function。

第 5 行

let calculate = mapSquare >> filterOdd >> sum

將 mapSquare()、filterOdd() 與 filterOdd() 組合成 calculate() 。

因為 mapSquare()、filterOdd() 與 sum() 的最後一個 argument 都是 list : 'T list，在 function composition 時，F# 允許省略之。

>> 為 F# 的 function composition，由左至右

我們可以發現 F# 在設計 function 時，會 故意 將要處理的 資料 放在最後一個 argument，將 條件 放在前面的 argument，如此所有 function 無論要做 pipeline 或 function composition 時，都可省略最後一個 argument，使程式碼更簡潔。

Pipeline 與 function composition 講的其實是同一件事情，只是 F# 文化較喜歡使用 pipeline，而 Haskell 較愛用 function composition

當初以為這只是 F# 的 syntax sugar，後來在歐陽繼超的 前端函數式攻城指南 與 Haskell 趣學指南 這兩本書，才發現這是 FP 特有風格，稱為 point-free。

Definition

Point-free
Function 不特別將要處理的 data 放進參 argument，因此也不回傳處理過的 data，而是回傳 function，這有助於 function composition，也稱為 Tacit Programming

Q：為甚麼 point-free 能成立呢 ?

let fn arr = List.map (fun x -> x * x) arr

若原本 fn() 只帶一個 argument，相當於將 arr 傳入 List.map (fun x -> x * x)，並回傳處理過的結果。

let fn = List.map (fun x -> x * x) 

由於 F# 的 function 天生是 curry function，fn() 沒有 argument，= 左右將 arr 同時消去，就相當於回傳 List.map (fun x -> x * x) 。

所以一個 function 將 data 放在最後一個 argument 時，提不提供 data 都成立：

• 有提供 data 則回傳處理過的 data
• 不提供 data 則回傳 function

由於回傳是 function，特別適合做 function composition。

Q：為什麼稱為 point-free ?

Point 所指的就是傳入 data 的 argument，point-free 就是指 function 將 data 放在最後一個 argument，要使用時故意將最後一個 argumet 丟棄 (free)，則變成回傳 function，但若 data 不是最後一個 argument，則無法丟棄，因此也無法變成 function，也無法繼續再做 function composition。

Q：為什麼 point-free 適合做 function composition ?

Function composition 事實上來自於數學的 合成函數，也就是 fog(x) = f(g(x))，其中：

fog(x) = f(y)
y      = g(x)

也就是 g(x) 的 output 剛好為 f(y) 的 input，因此才能將 f(g(x)) 合併，變成 fog(x)，其中的 y 剛好被消滅。

若 f() 與 g() 每個 function 的 格式都一樣，都是最後一個 argument 為 data，則可將所有 function 加以組合成一個新 function，這就是 function composition。

ECMAScript

前面談的都是 F#，你可能看得似懂非懂，我們就來將相同程式碼以大家熟悉的 ECMAScript 改寫：

Pipeline

let map = fn => arr => arr.map(fn)
let filter = fn => arr => arr.filter(fn)
let reduce = fn => arr => arr.reduce(fn)

let mapSquare = map(x => x * x)
let filterOdd = filter(x => x % 2)
let sum = reduce((a, x) => a + x)

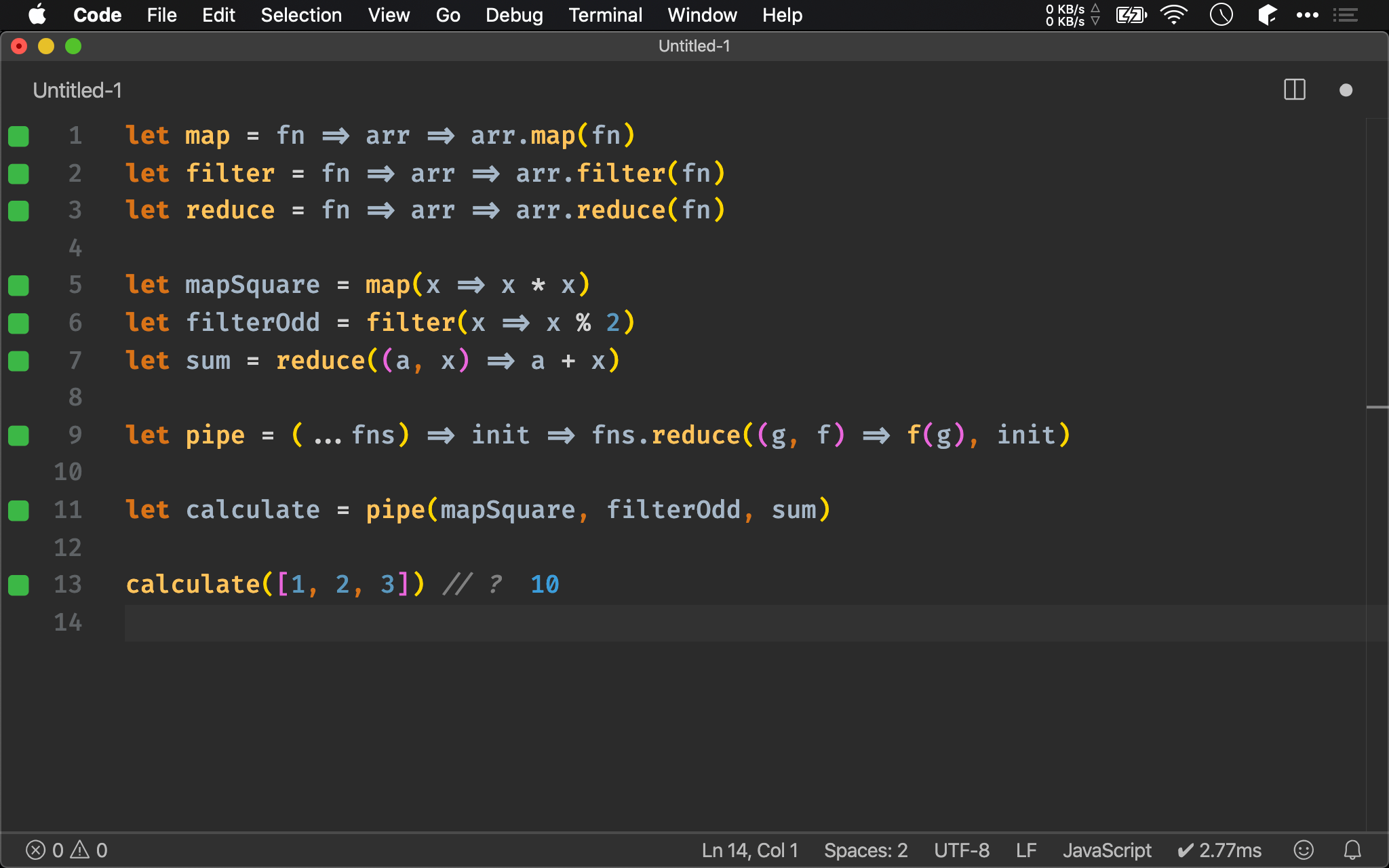
let pipe = (...fns) => init => fns.reduce((g, f) => f(g), init) 

let calculate = pipe(mapSquare, filterOdd, sum)

calculate([1, 2, 3]) // ?

第 1 行

let map = fn => arr => arr.map(fn)
let filter = fn => arr => arr.filter(fn)
let reduce = fn => arr => arr.reduce(fn)

ECMAScript 雖然都有提供 map()、filter() 與 reduce()，但這些都不是 curry function，因此無法使用 function composition，所以第一步就是將這些 function 改寫成 curry function。

第 5 行

let mapSquare = map(x => x * x)
let filterOdd = filter(x => x % 2)
let sum = reduce((a, x) => a + x)

改寫成 point-free function。

第 9 行

let pipe = (...fns) => init => fns.reduce((g, f) => f(g), init) 

由於 ECMAScript 沒有提供 pipe() 組合 function，我們自己土炮用 reduce() 寫一個 pipe()，負責將多個 point-free function 組合成單一 function。

11 行

let calculate = pipe(mapSquare, filterOdd, sum)

將 mapSquare()、filterOdd() 與 sum() 組合成 calculate()。

這裡與 F# 一樣，而是 由左至右。

Function Composition

let map = fn => arr => arr.map(fn)
let filter = fn => arr => arr.filter(fn)
let reduce = fn => arr => arr.reduce(fn)

let mapSquare = map(x => x * x)
let filterOdd = filter(x => x % 2)
let sum = reduce((a, x) => a + x)

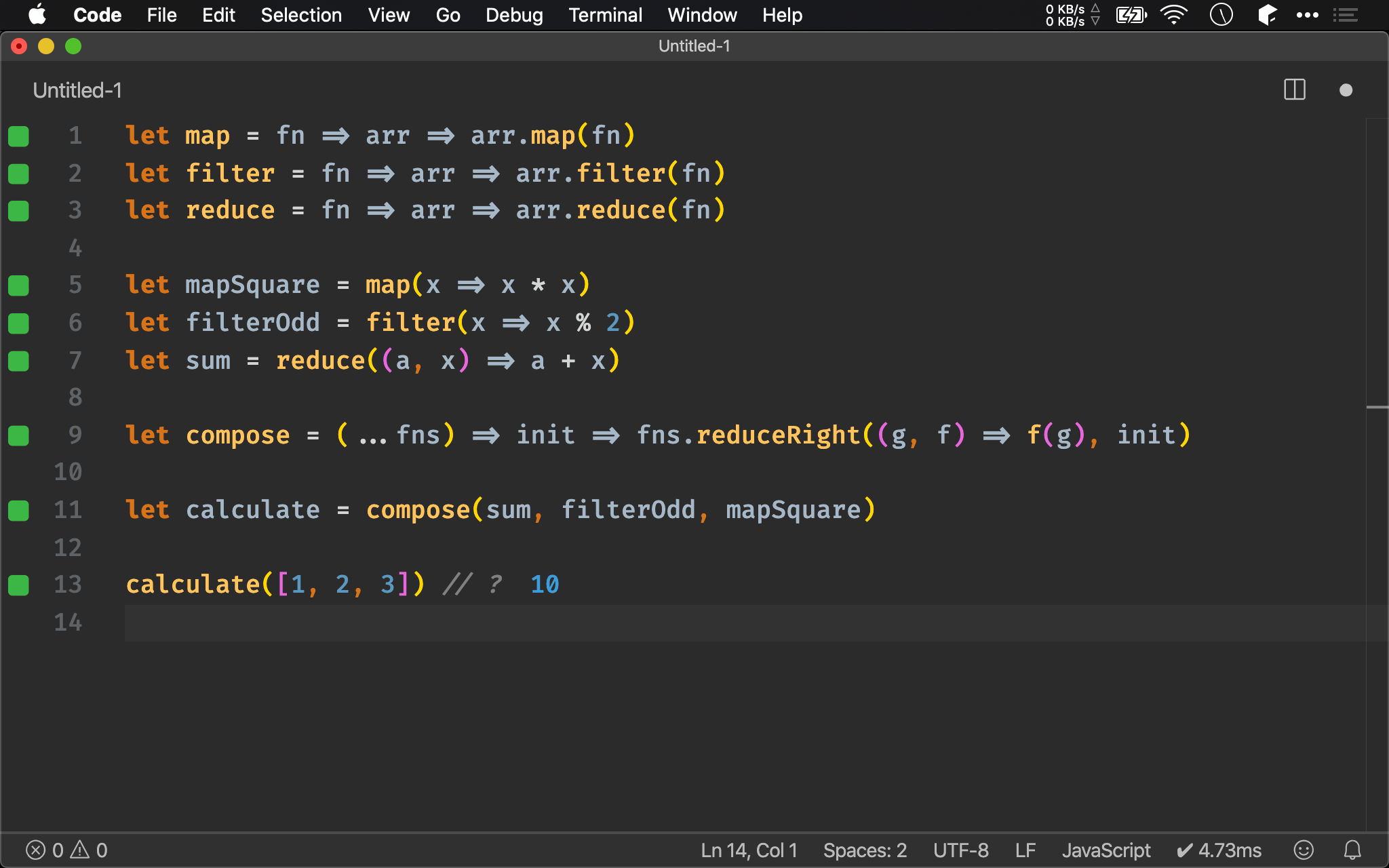
let compose = (...fns) => init => fns.reduceRight((g, f) => f(g), init)

let calculate = compose(sum, filterOdd, mapSquare)

calculate([1, 2, 3]) // ?

第 9 行

let compose = (...fns) => init => fns.reduceRight((g, f) => f(g), init)

由於 ECMAScript 沒有提供 compose() 組合 function，我們自己土炮用 reduceRight() 寫一個 compose()，負責將多個 point-free function 組合成單一 function。

11 行

let calculate = compose(sum, filterOdd, mapSquare)

將 mapSquare()、filterOdd() 與 sum() 組合成 calculate()。

這裡與 F# 不一樣，而是 由右至左。

ECMAScript 雖然寫的出來，但由於沒有直接支援 curry function 、pipe() 與 compose()，因此寫起來有些冗長。

Ramda

Pipeline

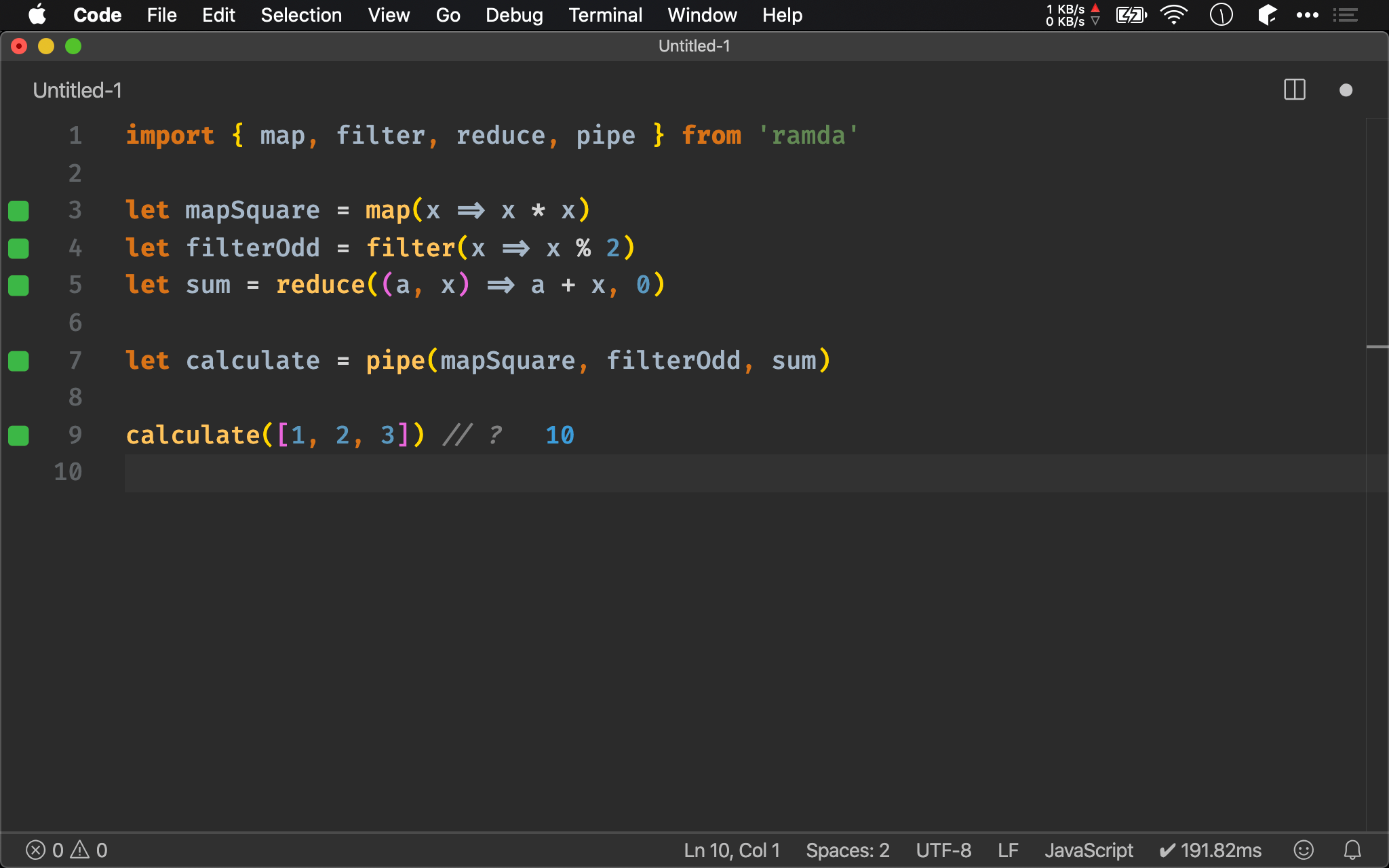
import { map, filter, reduce, pipe } from 'ramda'

let mapSquare = map(x => x * x)
let filterOdd = filter(x => x % 2)
let sum = reduce((a, x) => a + x, 0)

let calculate = pipe(mapSquare, filterOdd, sum)

calculate([1, 2, 3]) // ? 

第 1 行

import { map, filter, reduce, pipe } from 'ramda'

從 Ramda 引入 map()、filter()、reduce() ，這些都是已經是 curry function。

也從 Ramda 引入 pipe()。

第 3 行

let mapSquare = map(x => x * x)
let filterOdd = filter(x => x % 2)
let sum = reduce((a, x) => a + x, 0)

使用 Ramda 的 function 改寫成 point-free function。

第 7 行

let calculate = pipe(mapSquare, filterOdd, sum)

使用 Ramda 的 pipe() 將 mapSquare()、filterOdd() 與 sum() 組合成 calculate()。

Function Composition

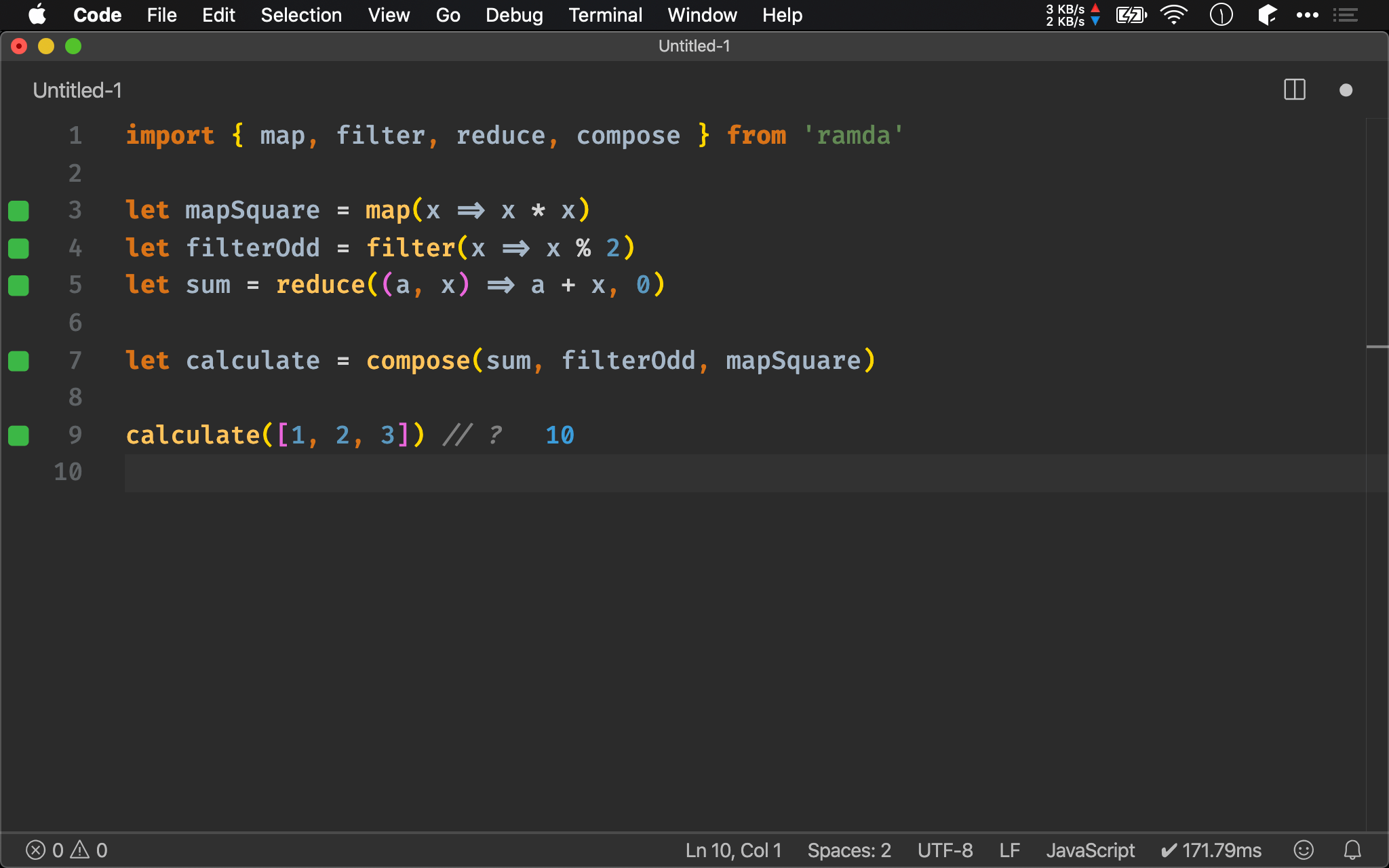
import { map, filter, reduce, compose } from 'ramda'

let mapSquare = map(x => x * x)
let filterOdd = filter(x => x % 2)
let sum = reduce((a, x) => a + x, 0)

let calculate = compose(sum, filterOdd, mapSquare)

calculate([1, 2, 3]) // ? 

第 1 行

import { map, filter, reduce, compose } from 'ramda'

從 Ramda 引入 map()、filter()、reduce() ，這些都是已經是 curry function。

也從 Ramda 引入 compose()。

第 7 行

let calculate = compose(sum, filterOdd, mapSquare)

使用 Ramda 的 compose() 將 mapSquare()、filterOdd() 與 sum() 組合成 calculate()。

Ramda 版本已經非常精簡，我們只需實作 point-free function 再加以組合即可，整體風格已經與純 FP 的 F# 非常接近。

Conclusion

• Point-free 是 FP 設計 argument 的基本精神，這也是為什麼歐陽繼超在前端函數式攻城指南一書中指出 Underscore 設計錯誤，因為 Underscore 是 _.map([1, 2, 3], x => x + 1)，將 data 放在第 1 個 argument，這並不符合 point-free 原則，因此 Underscore 無法使用 function composition
• FP 首重觀念，只要心裡有 function composition，無論是在 F# 或在 ECMAScript 都可實作
• 純 ECMAScript 實作稍微冗長，若使用 Ramda，則整體精簡程度已經與 F# 非常接近

Reference

歐陽繼超，前端函數式攻城指南
Miran Lipovaca, Haskell 趣學指南
Wikipedia, Tacit Programming